Mesh Adaptivity

Overview

Simmetrix' mesh adaptation technology enables adaptive refinement and coarsening of meshes. The mesh may be adapted to conform to either an isotropic or anisotropic size field such as might be obtained from an error indicator or estimator. As with mesh generation, the adapted mesh remains fully associative with the geometric model and conforms to the geometry boundaries.

Mesh adaptivity allows you to run fully automatic, in-memory adaptive simulations with a guaranteed geometry conforming mesh. Mesh adaptivity can be used to both improve solution accuracy and to ensure the mesh follows solution features in a time-dependent solution.

Capabilities:

• Unstructured adaptation of tetrahedral volume (and triangular surface) meshes.
• Structured adaptation of prismatic 3D (and quadrilateral 2D) boundary layer elements.
• Boundary layer mesh can be adapted both in-plane and in the thickness direction.
• Fully automatic in-place solution transfer as the mesh is being adapted.
• Adaptation of moving, evolving meshes.
• Automatic maintenance of periodicity (mesh matching) during adaptation.
• Efficient local adaptation when affecting a small portion of the mesh.
• Mesh gradation control during adaptation.
• Mesh adaptation to attributes specified on the geometric model, similar to initial mesh generation.
• Distributed and multithreaded parallel support with automatic, predictive load balancing.

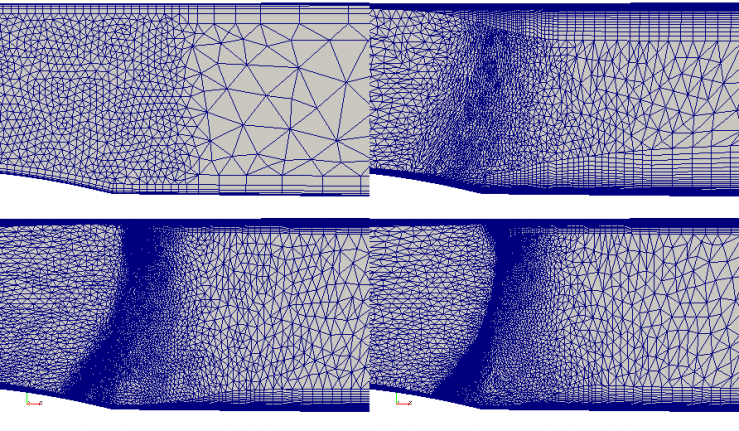
Boundary Layer Adaptation

Transonic flow in a channel encounters a bump (partly seen at the bottom left of the images) and becomes supersonic leading to a shock which is captured by adapting the unstructured mesh as well as the semistructured boundary layer mesh in both inplane and thickness directions.

Mesh Adaptation Based on Analytic Function Feedback

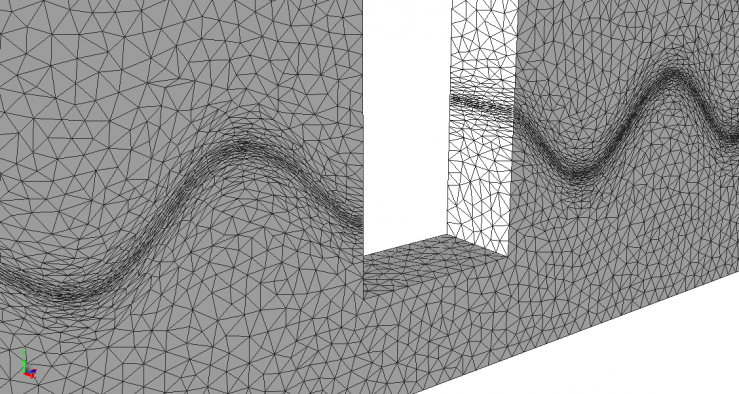
MeshSim Adapt can be driven by any source of information that can provide new sizes as a function of position in the domain. This animation shows a simple demonstration of using anisotropic adaptivity to track an internal interface within the mesh. Here the interface is given by an analytic function representing a wave moving through the domain. At each, step the mesh is adapted to correspond to the new position of the wave (the frequency of mesh adaptation can be controlled).

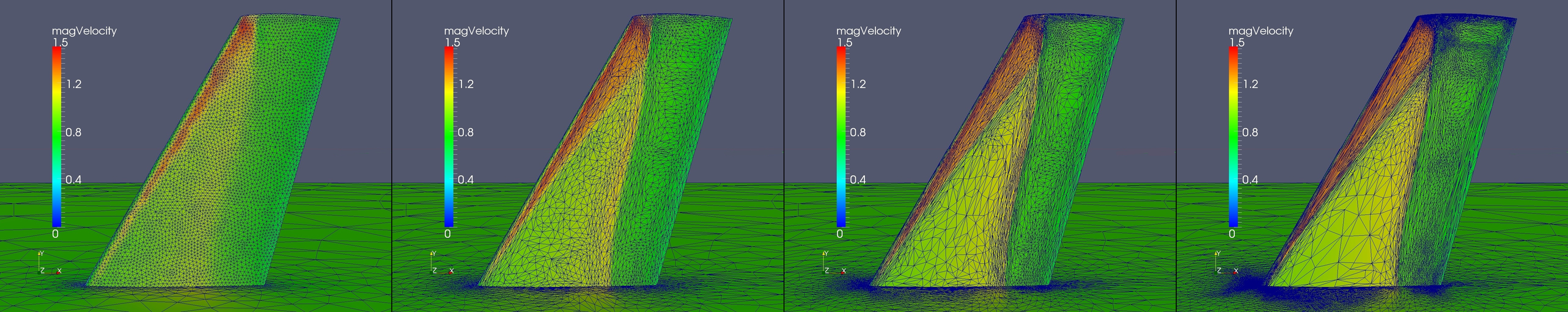
Evolving Domain Adaptation